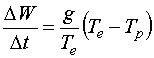
Homework Questions for Chapter 10 - Wind: Small-scale and local systems
Questions 1 and 2 will be turned in for a grade. Questions 3-11 will be discussed by the discussion groups in class so please look them over before the discussion session.
SHOW ALL WORK , CIRCLE THE CORRECT ANSWER, PLEASE BE NEAT AND STAPLE YOUR HOMEWORK!
ALSO, PLEASE USE A SPREADSHEET FOR ALL GRAPHS
Follow the Problem solving steps discussed in class
1. One of the primary forces that generate thermals in the boundary layer is buoyancy (a warm air parcel rising within a cooler environment). The resultant acceleration experience by a thermal due to buoyancy can be expressed as:
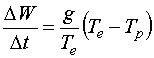
where DW is the change in vertical velocity over a period of time, Dt and Te and Tp are the environmental and parcel temperatures, respectively. If the parcel temperature is 10% larger than that of the environment, how long will it take for the thermals vertical velocity to reach a value of 3 ms-1? Assume that at the initial time, the parcel is at rest.
2. Wind drag at the surface depends strongly on the surface roughness. For example, a sparse forest creates more drag than a smooth snow-covered surface. An aerodynamic roughness length Z0 quantifies this effect. Values for different landscape types are given below:
Z0 (m) |
Classification |
Landscape |
| 0.0002 | sea | sea, paved areas, snow-covered flat plain, tide flast, smooth desert |
| 0.03 | open | grass prairie or farm fields, tundras, airports, heather |
| 0.25 | rough | high crops, crops of varied height, scattered obstacles such as trees or hedge-rows, vineyards |
| 1.0 | closed | regular coverage with large size obstacles with open spaces rouphly equal to obstacle heights, suburban houses, villages, mature forests |
The wind speed (WS) is defined to be zero at the ground (more precisely, at the height equal to the aerodynamic roughness length). The wind speed near the surface depends upon the roughness length according the the following relationship:
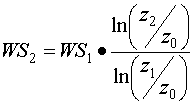
For example, if you know the wind speed WS1 at a height of Z1, then you can calculate the wind speed WS2 at any other height Z2.
a. Make a plot of height (z) up to 150 meters versus wind speed here at LSC given a wind observation of 5 ms-1 at a height of 10 m. Be sure to use the data given in the table above.
b. If you were in the business of producing energy from wind with a wind turbine, at what altitude(s) would you place your turbine for maximum energy production? HINT: Consider two factors. The benefit of building a very tall tower to tap into stronger winds versus the increased cost of taller towers. So, at what altitude would you build a tall tower to maximize energy production, yet, the cost of building the tower is not prohibitive.
3. A pilot enters the weather service office and wants to know what time of the day she can expect to encounter the least turbulent winds at 760 m above central Kansas. If you were the weather forecaster, what would you tell her?
4. Why is it dangerous during hang gliding to enter the leeward side of the hill when the wind speed is strong?
5. After a winter snowstorm, Cheyenne, Wyoming reports a total snow accumulation of 48 cm, while the maximum depth in the surrounding countryside is only 28 cm. If the storms intensity and duration were practically the same for a radius of 50 km around Cheyenne, explain why Cheyenne received so much more snow.
6. Why is the difference in surface wind speed between morning and afternoon typically greater on a clear, sunny day than on a cloudy, overcast day?
7. Explain why cities near large bodies of cold water in summer experience well-developed sea breeezes, but only poorly-developed land breezes.
8. If campfire smoke is blowing uphill along the east-facing side of the hill and downhill along the west-facing side of the same hill, are the fires cooking breakfast or dinner? From the drift of the smoke, how were you able to tell?
9. Why don't chinook winds form on the east side of the Appalachians?
10. Show, with the aid of a diagram, what atmospheric and topographic conditions are necessary for an area in the Northern Hemisphere to experience hot summer breezes from the north.
11. The prevailing winds in southern Florida are northeasterly. Knowing this, would you expect the strongest sea breezes to be along the east or west coast of southern Florida? What about the strongest land breezes.
EXTRA CREDIT: When stable air flows over a hill or mountain, mountain waves develop in the flow with wavelength, l. The waves are often visible in satellite imagery. There are two mechanisms that damp the waves with time; they are friction and turbulence. Hence, the vertical displacement of a parcel of air moving over the mountain can be expressed as:
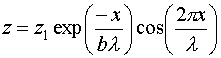
where Z is the height of the air above its starting equilibrium height, Z1 is the initial amplitude of the wave (based on the height of the mountain), x is distance downwind of the mountain crest, and b is a damping factor.
a. At what distances, x, does the parcel height decrease by a factor of e-n, where n is a positive integer?
b. Make a plot of Z versus x for a wavelength of 15 km and a damping factor of 3. Assume that the initial wave amplitude is 500m.